fmod.c File Reference

Return x mod y in exact arithmetic. More...

#include "prim.h"

Include dependency graph for fmod.c:

Go to the source code of this file.

Functions
double	fmod (double x, double y)
	Return x mod y in exact arithmetic. More...

Variables
static const double	one = 1.0
static const double	Zero []

Detailed Description

Return x mod y in exact arithmetic.

Definition in file fmod.c.

Function Documentation

fmod()

double fmod	(	double	x,
		double	y
	)

Return x mod y in exact arithmetic.

Method: Shift and subtract

Definition at line 58 of file fmod.c.

References one, and Zero.

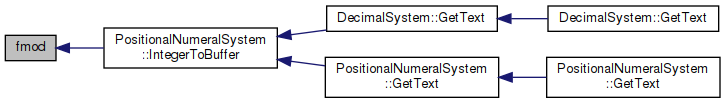
Referenced by PositionalNumeralSystem::IntegerToBuffer().

{
    int32_t n, hx, hy, hz, ix, iy, sx, i;
    uint32_t lx, ly, lz;

    EXTRACT_WORDS(hx, lx, x);
    EXTRACT_WORDS(hy, ly, y);
    sx = hx & 0x80000000; /* sign of x */
    hx ^= sx;             /* |x| */
    hy &= 0x7fffffff;     /* |y| */

    /* purge off exception values */
    if ((hy | ly) == 0 || (hx >= 0x7ff00000) ||   /* y=0,or x not finite */
        ((hy | ((ly | -ly) >> 31)) > 0x7ff00000)) /* or y is NaN */
        return (x * y) / (x * y);
    if (hx <= hy)
    {
        if ((hx < hy) || (lx < ly))
            return x; /* |x|<|y| return x */
        if (lx == ly)
            return Zero[(uint32_t)sx >> 31]; /* |x|=|y| return x*0*/
    }

    /* determine ix = ilogb(x) */
    if (hx < 0x00100000)
    { /* subnormal x */
        if (hx == 0)
        {
            for (ix = -1043, i = lx; i > 0; i <<= 1)
                ix -= 1;
        }
        else
        {
            for (ix = -1022, i = (hx << 11); i > 0; i <<= 1)
                ix -= 1;
        }
    }
    else
        ix = (hx >> 20) - 1023;

    /* determine iy = ilogb(y) */
    if (hy < 0x00100000)
    { /* subnormal y */
        if (hy == 0)
        {
            for (iy = -1043, i = ly; i > 0; i <<= 1)
                iy -= 1;
        }
        else
        {
            for (iy = -1022, i = (hy << 11); i > 0; i <<= 1)
                iy -= 1;
        }
    }
    else
        iy = (hy >> 20) - 1023;

    /* set up {hx,lx}, {hy,ly} and align y to x */
    if (ix >= -1022)
        hx = 0x00100000 | (0x000fffff & hx);
    else
    { /* subnormal x, shift x to normal */
        n = -1022 - ix;
        if (n <= 31)
        {
            hx = (hx << n) | (lx >> (32 - n));
            lx <<= n;
        }
        else
        {
            hx = lx << (n - 32);
            lx = 0;
        }
    }
    if (iy >= -1022)
        hy = 0x00100000 | (0x000fffff & hy);
    else
    { /* subnormal y, shift y to normal */
        n = -1022 - iy;
        if (n <= 31)
        {
            hy = (hy << n) | (ly >> (32 - n));
            ly <<= n;
        }
        else
        {
            hy = ly << (n - 32);
            ly = 0;
        }
    }

    /* fix point fmod */
    n = ix - iy;
    while (n--)
    {
        hz = hx - hy;
        lz = lx - ly;
        if (lx < ly)
            hz -= 1;
        if (hz < 0)
        {
            hx = hx + hx + (lx >> 31);
            lx = lx + lx;
        }
        else
        {
            if ((hz | lz) == 0) /* return sign(x)*0 */
                return Zero[(uint32_t)sx >> 31];
            hx = hz + hz + (lz >> 31);
            lx = lz + lz;
        }
    }
    hz = hx - hy;
    lz = lx - ly;
    if (lx < ly)
        hz -= 1;
    if (hz >= 0)
    {
        hx = hz;
        lx = lz;
    }

    /* convert back to floating value and restore the sign */
    if ((hx | lx) == 0) /* return sign(x)*0 */
        return Zero[(unsigned)sx >> 31];
    while (hx < 0x00100000)
    { /* normalize x */
        hx = hx + hx + (lx >> 31);
        lx = lx + lx;
        iy -= 1;
    }
    if (iy >= -1022)
    { /* normalize output */
        hx = ((hx - 0x00100000) | ((iy + 1023) << 20));
        INSERT_WORDS(x, hx | sx, lx);
    }
    else
    { /* subnormal output */
        n = -1022 - iy;
        if (n <= 20)
        {
            lx = (lx >> n) | ((uint32_t)hx << (32 - n));
            hx >>= n;
        }
        else if (n <= 31)
        {
            lx = (hx << (32 - n)) | (lx >> n);
            hx = sx;
        }
        else
        {
            lx = hx >> (n - 32);
            hx = sx;
        }
        INSERT_WORDS(x, hx | sx, lx);
        x *= one; /* create necessary signal */
    }
    return x; /* exact output */
}

Here is the caller graph for this function:

Variable Documentation

one

const double one = 1.0

static

Definition at line 49 of file fmod.c.

Referenced by fmod().

Zero

const double Zero[]

static

Initial value:

= {
        0.0, -0.0,
}

Definition at line 50 of file fmod.c.

Referenced by fmod().